Answer (1 of 5) 1st there is ALWAYS an Odd leg & a Quad doubly even leg in a primitive triplet 7, 24, 25;Solution False 3, 4 and 5 are the numbers of the Pythagorean triplet as 52 =4232 where 4 is not an odd number Suggest corrections2 Generating All Pythagorean Triples When asked to give examples of Pythagorean triples, a typical math student can usually give two or three examples (3,4,5), (5,12,13) and maybe (15,8,17) Not many students can come up with more triples o the top of their heads It is therefore desirable to nd a way of generating Pythagorean triples that is

Pythagorean Triples
3 4 5 is a pythagorean triplet true or false
3 4 5 is a pythagorean triplet true or false-Answer (1 of 3) Pythagorean triplets are obtained by finding AP series of each number with same number as its common difference Like 3, 4, 5 is the first tripletA true B false
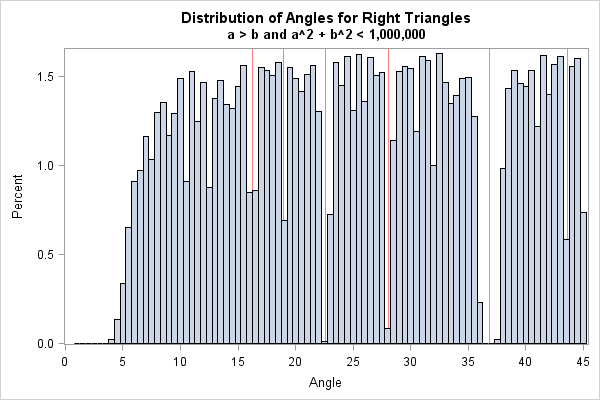



The Distribution Of Pythagorean Triples By Angle The Do Loop
Start studying M2M6 Geometry Learn vocabulary, terms, and more with flashcards, games, and other study toolsA in 3 in B C36 in 6 in In the figure shown, LQSR is a right angle True or false? iii 5 iv 2, 3, 7, 8 v 5 Question 2 Say True or False i When a square number ends in 6, its square root will have 6 in the unit's place ii A square number will not have odd number of zeros at the end iii The number of zeros in the square of is 9 iv (7, 24, 25) is a Pythagorean triplet v The square root of 221 is 21
M 21=41=5 ∴(3,4,5) is a Pythagorean triplet (ii) (10,24,26) 2m=10 ⇒m=5 m 2−1=25−1=24 m 21=251=26 ∴(10,24,26) is not a Pythagorean triplet (iii) (6,7,8)16 Each leg of an isosceles right triangle This problem has Cycles #3, #4, #5 of the "Discrete Pythagorean Theorem" with a rational C² Pythagorean Triples Everyone loves the Pythagorean
Answer (1 of 7) In the above given figure, tiangle ABC has sides AC = 5unit, AB = 4 unit, BC = 3 unit And,since, 5² = 4² 3² So, AC² = AB² BC² TO SHOW THAT with these given measures, the triangle ABC can not be without a right angle Here, just by the side, i constructed a right triangleWrite a C program that checks if a set of three integers form a Pythagorean triple, ie they satisfys ܽ ଶ ܾ ଶ ൌ ܿ ଶ Refer to the following samples Sample 1 Enter three integers 1 2 3 This is not a Pythagorean triple Sample 2 Enter three integers 3 4 5 This is a Pythagorean tripleA true B false In the figure shown, LJLK is a right angle True or false?




Pythagorean Triples List Examples Definition Video Tutors Com
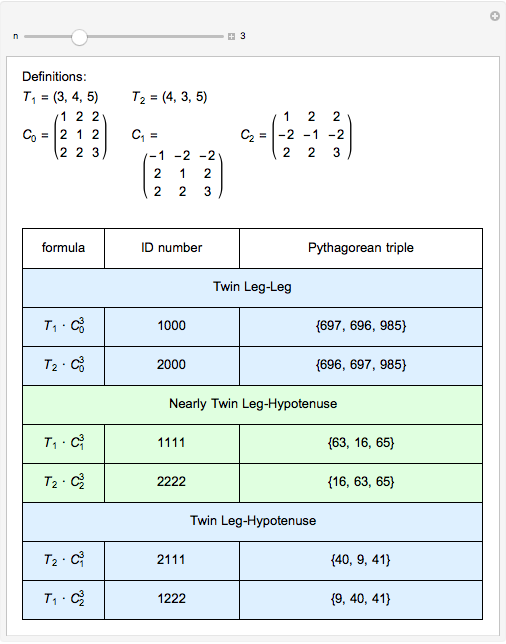



Twin And Nearly Twin Pythagorean Triples Wolfram Demonstrations Project
Pythagorean Triplet Given an array arr of N integers, write a function that returns true if there is a triplet (a, b, c) that satisfies a2 b2 = c2, otherwise false Input N = 5 Arr = {3, 2, 4, 6, 5} Output Yes Explanation a=3, b=4, and c=5 forms a pythagorean triplet16 8√2 500 The length of the long leg is 8√3True 2k, (k2 1) and (k2 −1) form a Pythagorean triplet for any number, k > 1 Let 2k = 16 Hence, k = 8 and, k2 1= 1 =641= 65 and, k2 −1= −1 =64−1= 63 Check the answers 632




Ex 6 2 2 I Write A Pythagorean Triplet Whose One Member Is 6




Pythagorean Theorem An Overview Sciencedirect Topics
The most famous Pythagorean triple of all is 3,4,5, and another is 5,12,13 In these two examples, c=b1, and there are many more examples of this type Take any number k, and put (an algebraic proof of this is given at the end of this article) For example,A6, 8, 12 b 3, 4, 5 c 1, 2, 3 d 5, 7, 12 Which of the following remains an unsolved problem in mathematics?A true B false A Pythagorean triple is a set of numbers that always satisfy the equation, a2 b2 = c2 The numbers, 30, 72, 78, are a Pythagorean triple True or false?




State True Or False Pythagorean Triplet Whose One Member Is 14 Is 14 48 50
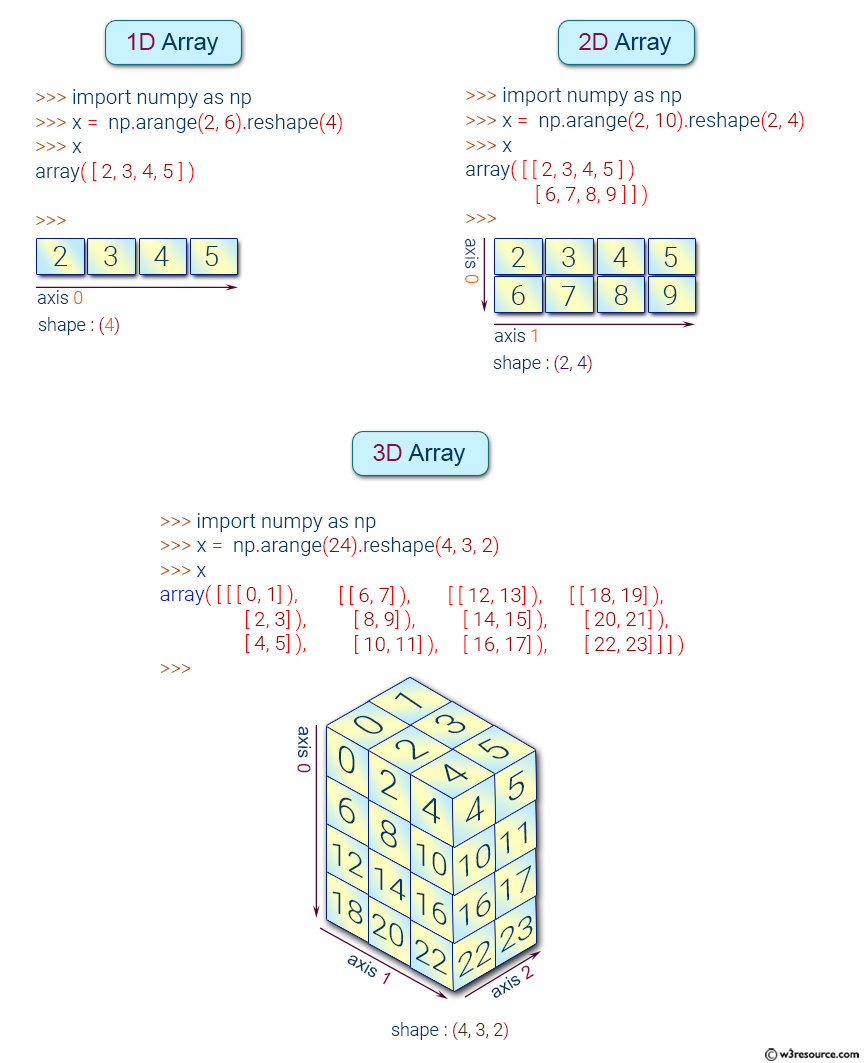



Numpy Array Object Exercises Practice Solution W3resource
Saksham1405 As we know that in a Pythagorean triplet the sum of the squares of two numbers is equal to the square of the third number So in this case square of 6 ie36 and square of 8 ie64 is equal to the square of 10 ie 100 so it is a Pythagorean triplet kaypeeoh72z and 3 more users found this answer helpful heart outlinedIn examples 15 to 19, state whether the statements are true (T) or false (F) Example 15 The square of 04 is 016 Solution True Example 16 The cube root of 729 is 8 Example 24 Write a pythagorean triplet whose smallest number is 6 Solution Smallest number is 6 2m = 6 or m = 3 m2 1 = 32 1 = 9 1 = 10 Find an answer to your question the numbers 45 28 53 form a pythagorean triple true or false Lauriall5amil6e Lauriall5amil6e Mathematics High School answered The numbers 45 28 53 form a pythagorean triple true or false 2 5 In predatorprey relationships, the populations of the predator and prey are often cyclical In a




Please Give This Fill In The Blanks Answer And True False Answer A Ng With 2 3 7 Or Maths Squares And Square Roots Meritnation Com



Pdf Pythagorean Triples Before And After Pythagoras
Yes A pythagorean triple is a sequence of integer numbers that solve the Pythagora's theorem It means that three numbers a,b,c are a pythagorean triples when √a2 b2 = c or, just to remove the square root and write it in a more elegant format a2 b2 = c2 With 3,4,5 we have thatSide length c represents the hypotenuse?Answer (1 of 3) Thanks for the A I'll give as comprehensive an answer as I can 1) If a^2b^2=c^2 and h is the length of the altitude to the hypotenuse then the area of the triangle is given by both \frac{ab}2 and \frac{ch}2 Equating these




B Show That 6 8 10 Is A Pythagorean Triplet Brainly In




Three Numbers Form A Pythagorean Triplet Two Of Them Are 15 And 17 Where 17 Is The Largest Of Them The Third Number Isa 8b 12c 13d 5correct Answer Is Option A Can You Explain This



0 件のコメント:
コメントを投稿